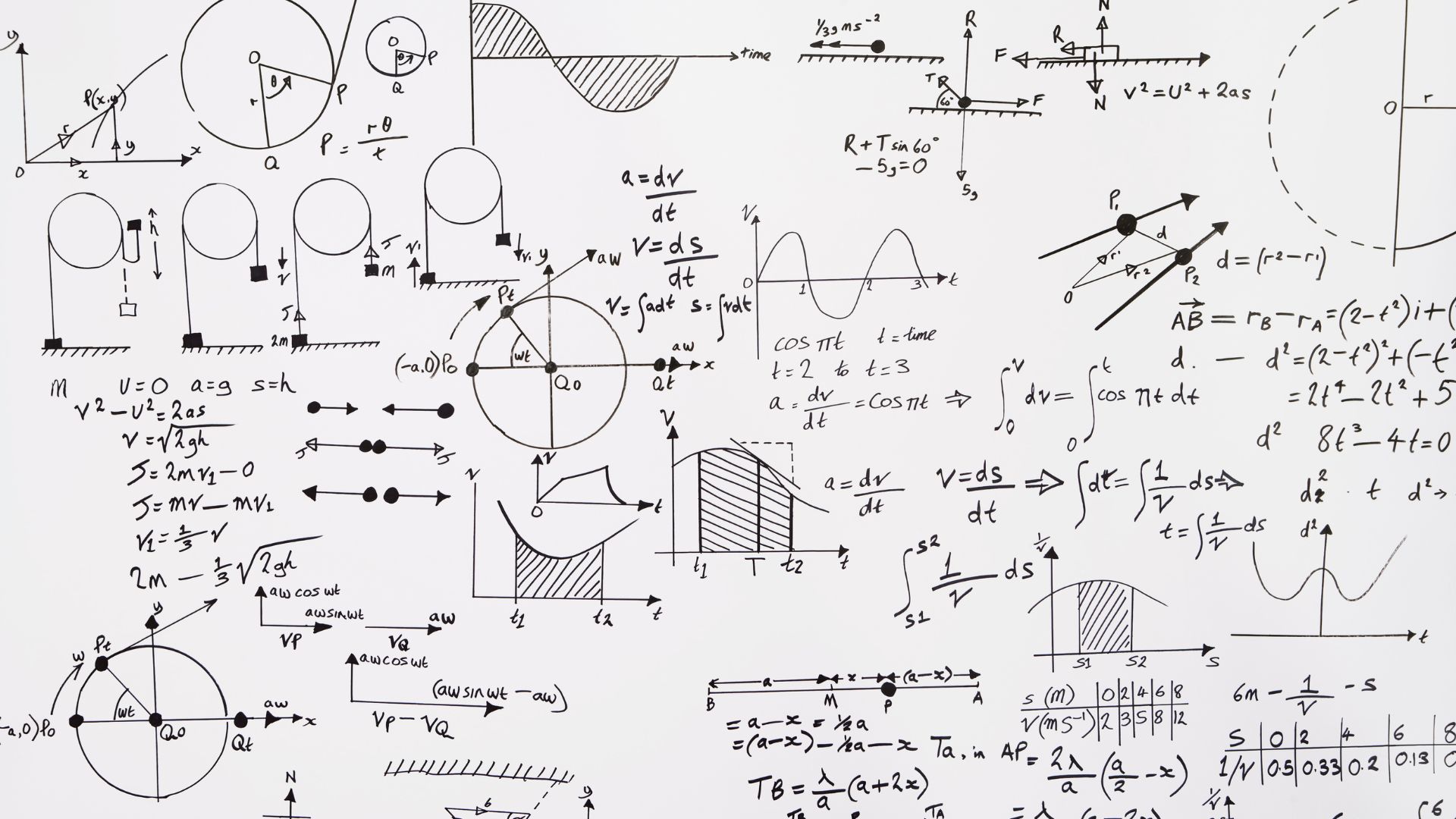
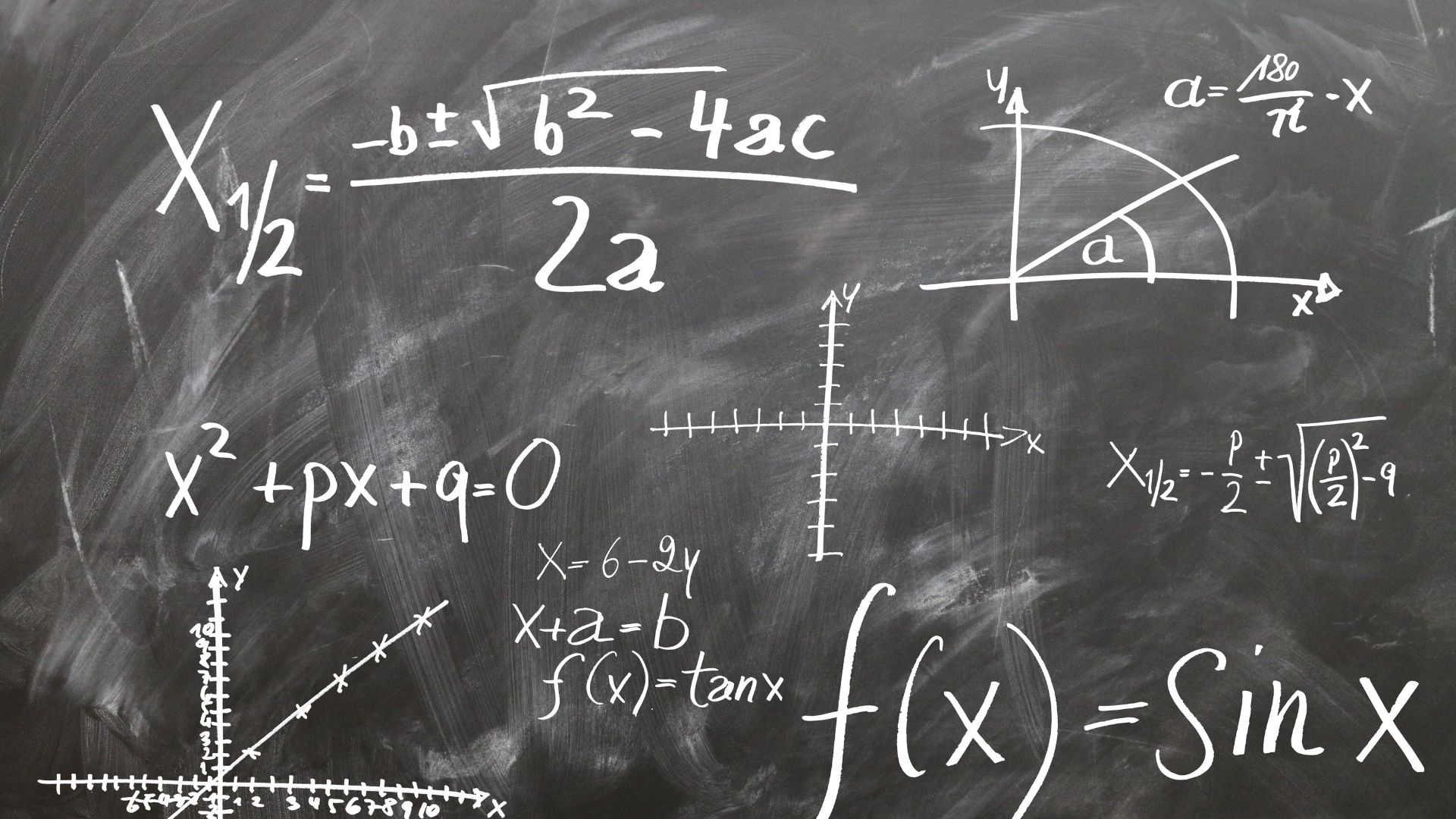
Differential Equations Demystified: Real-World Examples and Tutorials for Understanding Their Impact

Differential equations, often seen as daunting, are fundamental tools in mathematics that describe how quantities change over time. Their importance spans various fields, from engineering and physics to biology and economics, making them essential for modeling dynamic systems.

To demystify differential equations, it is helpful to begin with a practical example: population dynamics. The classic logistic growth model, represented by a differential equation, describes how populations grow in an environment with limited resources. The equation illustrates that growth slows as the population approaches the carrying capacity of its environment. This understanding has implications for ecology, conservation efforts, and resource management.

Another significant application of differential equations is in engineering, particularly in the analysis of electrical circuits. Kirchhoff’s laws, when translated into differential equations, allow engineers to model the behavior of circuits over time. By solving these equations, engineers can predict how currents and voltages change, enabling the design of more efficient and reliable electronic devices.

In fluid dynamics, differential equations govern the behavior of fluids in motion. The Navier-Stokes equations, a set of nonlinear partial differential equations, describe how velocity and pressure fields interact in a fluid. Understanding these equations is crucial for applications such as aerodynamics, where engineers must predict airflow over aircraft wings to optimize performance and reduce drag.

For those looking to understand differential equations better, numerous online resources and tutorials are available. Websites like Khan Academy and Coursera offer courses that break down complex concepts into manageable lessons. These platforms often include interactive exercises that allow learners to practice solving differential equations in a guided manner, reinforcing their understanding through application.

Moreover, software tools such as MATLAB and Mathematica are invaluable for both solving and visualizing differential equations. These tools enable students and professionals to model real-world phenomena, making the abstract concepts more tangible. By utilizing graphical representations, individuals can see the behavior of solutions over time, gaining insights that purely numerical approaches might miss.

In conclusion, differential equations are powerful mathematical tools that unlock insights into the behavior of dynamic systems. By bridging the gap between theory and application, they empower engineers, scientists, and students to tackle real-world challenges. Understanding and mastering these equations is essential for anyone looking to innovate and contribute to their respective fields.